Space figure
A space figure is a geometric figure that occupies space and has volume. They are also referred to as three-dimensional (3D) figures. Space figures are the 3D counterpart to plane figures, which are two-dimensional (2D) figures.
Unlike 2D figures which only have two dimensions, such as length and width, space figures have the additional dimension of height (or depth), which results in them taking up space, and thus having volume.
Types of space figures
There are many different types and of space figures. Below are a few examples:
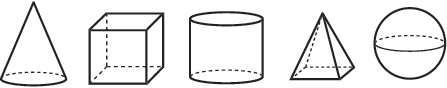
From left to right, the figures above are a cone, cube, cylinder, pyramid, and sphere. Certain space figures can be categorized, such as the pyramid and cube. Both the pyramid and cube are polyhedra, which are space figures that are made up of flat surfaces, referred to as faces, that are polygons.
Prisms are another category of space figure. The cube above can also be classified as a rectangular prism. A prism is a space figure that has two identical polygon faces that are connected by rectangular faces.
Other space figures, such as spheres, cylinders, and cones, do not easily fit into other categories, and are typically discussed individually. There are many more space figures than those described above, but these are some of the most common.
2D and 3D figures
As mentioned above, space figures are the 3D counterpart of plane figures. It follows that space figures, such as those above, are made up of 2D figures.
- Cone - a cone is constructed by rotating a right triangle about either of the legs adjacent to the right angle.
- Cube - a cube is just 6 interconnected square faces.
- Cylinder - a cylinder can be formed by rotating a rectangle around one of its sides.
- Pyramid - a square pyramid is made up of a square base and 4 triangular faces that meet at a point referred to as the apex of the pyramid.
- Sphere - a sphere can be formed by rotating a semicircle around its diameter.
Because 3D figures are formed using various 2D figures, calculations of volume are typically closely related to the area formulas of the corresponding 2D figures, with the addition of the third dimension of depth/height. Similarly, the surface area of a space figure is calculated as the sum of the areas of its faces. Refer to the volume and surface area section for more information on calculating the volume and surface area of various space figures.
