Plane symmetry

A space figure has plane symmetry if it can be divided into two halves by a plane and have each half be a reflection of the other across the plane. The plane is called a plane of symmetry of the space figure.

Slicing a round orange with a knife into two equal halves creates a plane of symmetry of the orange.

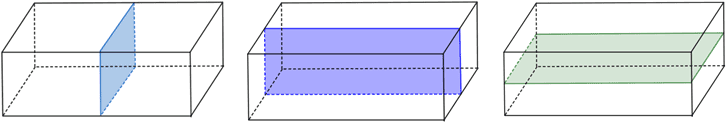
Not all planes of reflection are also planes of symmetry. Although the plane shown below cuts the rectangular prism into two equal halves, it is not a plane of symmetry. If we only took vertex A for the prism and reflected it across the plane, we can see that the image, A', is not mapped to the other half of the prism. This is true for all points that lie in the prism except the ones that intersect with the plane.

Multiple planes of symmetry

Geometric solids can have multiple planes of symmetry. For example, the rectangular prism below has three planes of symmetry.

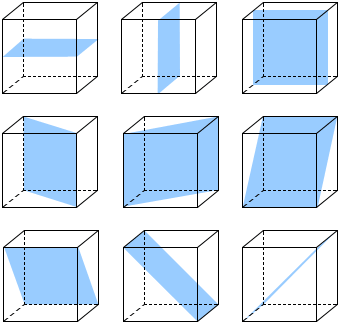
A cube has nine planes of symmetry. Three planes of symmetry are parallel to the surfaces and six planes of symmetry are diagonals.

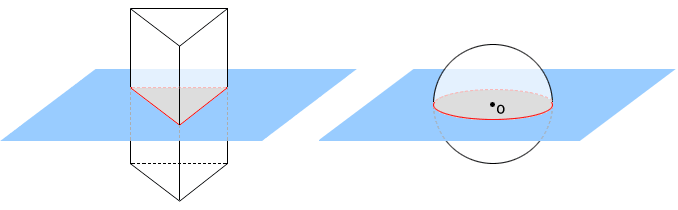
A sphere contains infinitely many planes of symmetry. If a plane contains the center point, O, of the sphere, it is a plane of symmetry for the sphere. Three of the infinitely many planes of symmetry are shown in the sphere below.