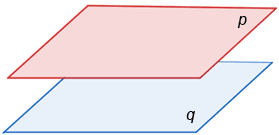
Parallel planes

Parallel planes are planes in space that never intersect.

Planes p and q do not intersect, so they are parallel.

Planes p and q intersect along line m, so they are not parallel.

Distance between parallel planes

The distance between two parallel planes is the length of any line segment from one plane to the other plane that is perpendicular to both planes.

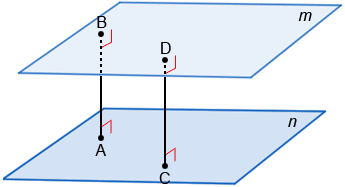
There are infinitely many line segments perpendicular to two parallel planes. Line segments AB and CD are two of those perpendicular segments shown above. The length of either line segment measures the distance between planes m and n. Any line segment perpendicular to both planes is also the shortest distance between the two planes.

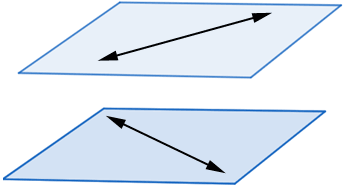
Lines in parallel planes

Lines in different parallel planes will never intersect since the parallel planes never intersect. However, just because two planes are parallel does not mean the lines contained in them must be parallel to each other. There are two types of lines in parallel planes: skew lines and parallel lines.

Skew lines don't intersect and are not parallel

Parallel lines in parallel planes.

Multiple parallel planes

If two planes are parallel to another plane, all three planes must be parallel.

Plane m is parallel to plane p and plane n is parallel to plane p, so by the transitive property, planes m and n are parallel to each other, making all three planes parallel.

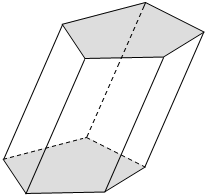
Geometric solids

Solid objects studied in geometry often have parallel surfaces.

If a polyhedron is a prism, it can be identified by its two congruent faces, called bases, that lie in parallel planes.

The pentagonal faces, called the bases, of the pentahedron above lie in parallel planes.

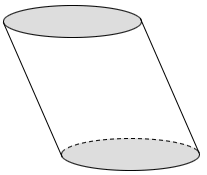
A cylinder is not a polyhedron, but it is a geometric solid whose bases lie in parallel planes.

The circular bases for the right cylinder above lie in parallel planes.