Union
In set theory, the union (∪) of a collection of sets is the set that contains all of the elements in the collection. For example, given two sets, A = {2, 2, 4, 6, 8, 10} and B = {1, 3, 5, 7, 9}, their union is as follows:
A ∪ B = {1, 2, 3, 4, 5, 6, 7, 8, 9 10}
Notice that even though A has two 2s, there is only one 2 in A ∪ B. This is because the union operation includes only one of each unique element.
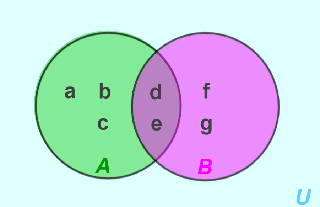
The union of two sets is commonly depicted using a Venn diagram, in which a set is represented by a circle. The Venn diagram below shows two sets: A = {a, b, c, d, e} and B = {d, e, f, g}. Their union is the set {a, b, c, d, e, f, g} and is represented in the Venn diagram by the area covered by both circles.
Properties of unions
Like other basic operations such as addition, set operations like unions also have certain properties. Refer to the set page if necessary for a table of symbols commonly used in set theory.
Unions and subsets
If set A is a subset of set B, then the union of the two sets is set B. Using set notation:
if A ⊆ B, then A ∪ B = B
For example, if A = {2n|n ∈ ℕ} and B is the set of integers, then A ∪ B = B, since set A is the set of positive even integers, which is a subset of all integers.
Commutative law
The commutative law states that the order in which the union of two sets is taken does not matter. Given two sets, A and B:
A ∪ B = B ∪ A
Let A = {1, 2, 3} and B = {3, 5, 7}. The sets share 1 common element. The union of the sets includes all unique elements of both sets. Thus, A ∪ B = B ∪ A = {1, 2, 3, 5, 7}. Regardless whether A or B is considered first, the result is the same. If B's elements were written first, the union of A and B could be written as {3, 5, 7, 1, 2}. The order in which elements are listed in a set does not matter; the number of elements and the values of the elements determine the set, so the above 2 sets are equal, as are any sets including all the same elements written in different orders.
Associative law
The associative law states that rearranging the parentheses in a union of sets does not change the result. Given sets A, B, and C:
(A ∪ B) ∪ C = A ∪ (B ∪ C)
Distributive law
For sets A, B, and C, the distributive law states
A ∪ (B ∩ C) = (A ∪ B) ∩ (A ∪ C)
A ∩ (B ∪ C) = (A ∩ B) ∪ (A ∩ C)
where A is distributed to B and C. This is similar to the distributive property of multiplication in which multiplication distributes over addition.
Example
Let A = {4, 6, 8, 10}, B = {8, 9, 10, 11}, and C = {10, 11, 12}. Show that A ∪ (B ∩ C) = (A ∪ B) ∩ (A ∪ C).
Compute the left side of the equation:
B ∩ C = {10, 11}
A ∪ (B ∩ C) = {4, 6, 8, 10} ∪ {10, 11} = {4, 6, 8, 10, 11}
Compute the right side of the equation:
A ∪ B = {4, 6, 8, 9, 10, 11}
A ∪ C = {4, 6, 8, 10, 11, 12}
(A ∪ B) ∩ (A ∪ C) = {4, 6, 8, 10, 11}
In both cases, the resulting set is {4, 6, 8, 10, 11}.
De Morgan's laws
In set theory, De Morgan's laws are a set of rules that relate the union and intersection of sets through their complements.
Union of sets:
The complement of the union of two sets is equal to the intersection of their complements:
(A ∪ B)C = AC ∩ BC
Given that A and B are subsets of the universal set 𝕌, this relationship can be seen in the figure below:

The union of A and B, A ∪ B, is shaded in blue. Its complement, (A ∪ B)C is shaded in yellow. The intersection of the complements of A and B, AC ∩ BC is also shaded in yellow.
Intersection of sets:
The complement of the intersection of two sets is equal to the union of their complements:
A ∩ B = AC ∪ BC
Given that A and B are subsets of the universal set 𝕌, this relationship can be seen in the figure below:

The intersection of A and B, A ∩ B, is shaded in red. Its complement, (A ∩ B)C is shaded in grey. The union of the complements of A and B, AC ∪ BC, is also shaded in grey.
